Data Visualization

• Graphical display of data

• Useful for

  • data cleaning
  • exploring data structure
  • detecting outliers and unusual groups
  • identifying trends and clusters
  • spotting local patterns
  • evaluating modeling output
  • Presenting results.

• Used to convey a message across, or to understand data better.
  

• A graphic won’t say the whole story.
  

• Presentation graphics are used to convey known information and are often designed to attract attention.

• Exploratory graphics are used to find new information and should direct attention to information.

Source - Why Is Data Visualization Important? What Is Important in Data Visualization?

1. Types

some below

Chart:

Source - Tableau.com

GeoSpatial

Source

Heatmaps

Bonus Point activity worth 2 points.- https://www.tableau.com/learn/articles/data-visualization#big-data

2. Good and Bad Vizualisation

Examples from : https://www.codeconquest.com/blog/12-bad-data-visualization-examples-explained/#htoc-what-does-this-graph-show

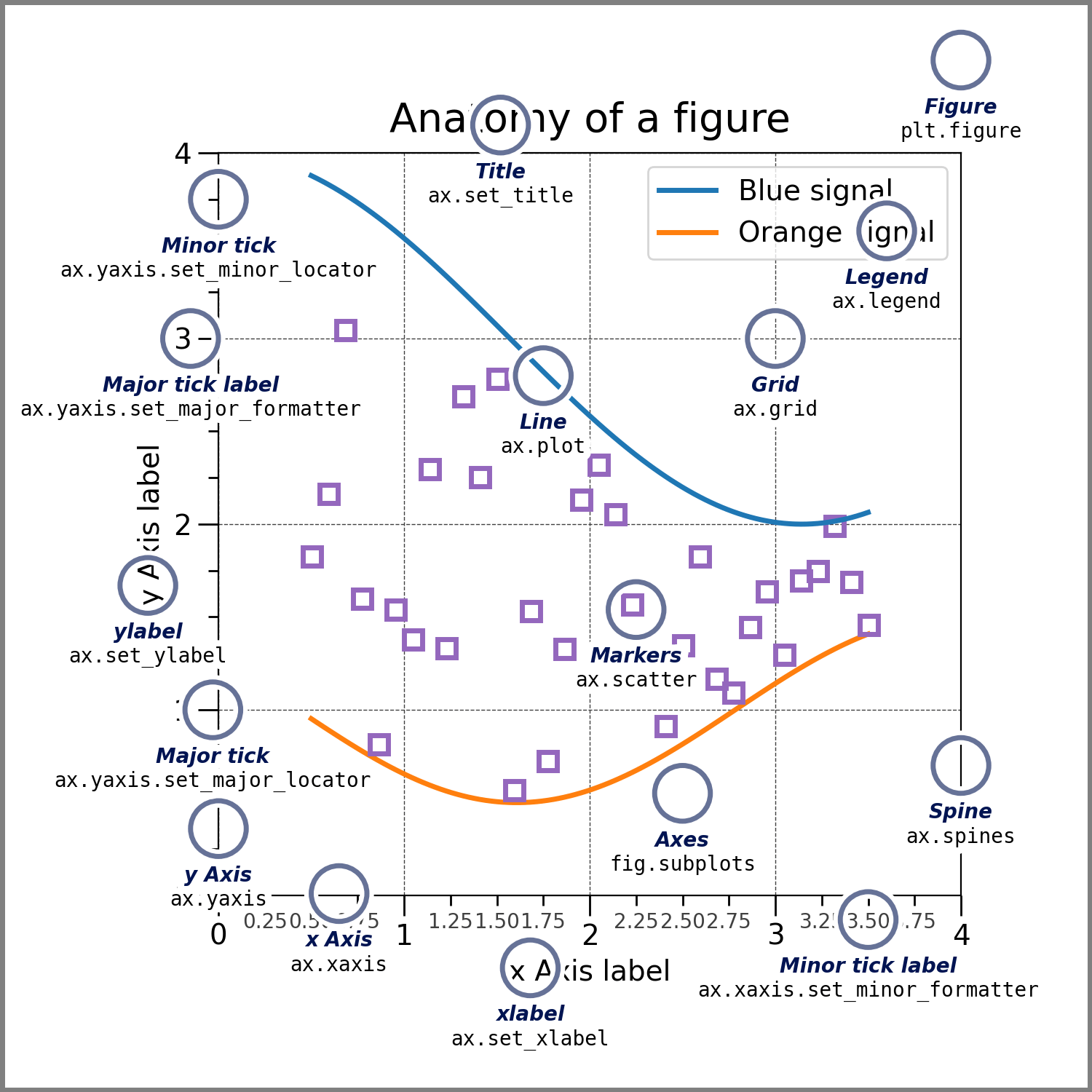
3. Key Principles of Effective Data Visualization

• Choosing the right visual representation for different types of data.
• Understanding the target audience and their needs.
• Designing for clarity, simplicity, and accuracy.
• Highlighting the main message and avoiding clutter.
• Using color, size, and shape effectively.

4. Python Libraries / Tools

• matplotlib
• plotly
• seaborn

Checkout -

• https://datavizcatalogue.com/search.html
• https://python-graph-gallery.com/

Matplotlib

Excellent Start - https://matplotlib.org/stable/tutorials/introductory/quick_start.html#

1. Plot linear, quadratic, and cubic functions from 0 to 2, with 100 equally spaced intervals

import matplotlib.pyplot as plt
import numpy as np

x = np.linspace(0, 2, 100)  # Sample data.
x

array([0.        , 0.02020202, 0.04040404, 0.06060606, 0.08080808,
       0.1010101 , 0.12121212, 0.14141414, 0.16161616, 0.18181818,
       0.2020202 , 0.22222222, 0.24242424, 0.26262626, 0.28282828,
       0.3030303 , 0.32323232, 0.34343434, 0.36363636, 0.38383838,
       0.4040404 , 0.42424242, 0.44444444, 0.46464646, 0.48484848,
       0.50505051, 0.52525253, 0.54545455, 0.56565657, 0.58585859,
       0.60606061, 0.62626263, 0.64646465, 0.66666667, 0.68686869,
       0.70707071, 0.72727273, 0.74747475, 0.76767677, 0.78787879,
       0.80808081, 0.82828283, 0.84848485, 0.86868687, 0.88888889,
       0.90909091, 0.92929293, 0.94949495, 0.96969697, 0.98989899,
       1.01010101, 1.03030303, 1.05050505, 1.07070707, 1.09090909,
       1.11111111, 1.13131313, 1.15151515, 1.17171717, 1.19191919,
       1.21212121, 1.23232323, 1.25252525, 1.27272727, 1.29292929,
       1.31313131, 1.33333333, 1.35353535, 1.37373737, 1.39393939,
       1.41414141, 1.43434343, 1.45454545, 1.47474747, 1.49494949,
       1.51515152, 1.53535354, 1.55555556, 1.57575758, 1.5959596 ,
       1.61616162, 1.63636364, 1.65656566, 1.67676768, 1.6969697 ,
       1.71717172, 1.73737374, 1.75757576, 1.77777778, 1.7979798 ,
       1.81818182, 1.83838384, 1.85858586, 1.87878788, 1.8989899 ,
       1.91919192, 1.93939394, 1.95959596, 1.97979798, 2.        ])

plt.figure(figsize=(5, 2.7), layout='constrained')

<Figure size 500x270 with 0 Axes>

<Figure size 500x270 with 0 Axes>

plt.figure(figsize=(5, 2.7), layout='constrained')
plt.plot(x, x, label='linear')  # Plot some data on the (implicit) axes.
plt.plot(x, x**2, label='quadratic')  # etc.
plt.plot(x, x**3, label='cubic')
plt.legend()

<matplotlib.legend.Legend at 0x117cadcc0>

plt.figure(figsize=(5, 2.7), layout='constrained')
plt.plot(x, x, label='linear')  # Plot some data on the (implicit) axes.
plt.plot(x, x**2, label='quadratic')  # etc.
plt.plot(x, x**3, label='cubic')
plt.xlabel('x label')
plt.ylabel('y label')
plt.title("Simple Plot")
plt.legend()
plt.show()

2. Multiple graphs in one figure

fig, axs = plt.subplots(2, 2, layout='constrained')
axs[0][0].plot(x,x)
axs[0][0].set_title("Linear")

axs[0][1].plot(x,x**2)
axs[0][1].set_xlabel("X Label")

axs[1][0].plot(x,x**3)
axs[1][0].set_ylabel("Y Label")
axs[1][1].plot(x,x**4, label="The Curve")
axs[1][1].legend()

plt.show()

fig, axd = plt.subplot_mosaic([['upleft', 'right'],
                               ['lowleft', 'right']], layout='constrained')
axd['upleft'].set_title('upleft')
axd['lowleft'].set_title('lowleft')
axd['right'].set_title('right')

Text(0.5, 1.0, 'right')